Ask a heating pro about the energy used by the systems they design or install. Their response is almost guaranteed to deal with “fuel” energy (e.g., natural gas, propane, oil, pellets, etc.) required by their systems.  

With the exception of systems with electrical boilers or heat pumps as their primary heat source, few heating pros give much thought to the electrical energy used in their systems. Perhaps they view such energy as trivial in comparison to fuel energy. They might regard it as simply a “parasitic” necessity for delivering heat produced by the high efficiency heat sources they specify or install.

That mindset has a historical parallel in the auto industry. Think back to what automobiles looked like in the mid-20th century. Many had large exterior “appurtenances” such as flat-facing mirrors, out-swinging vent windows, chrome trim, hood ornaments, massive front ends and other objects that were part of the auto persona of that time. A 1956 Cadillac Fleetwood weighed more than 5,000 pounds, about the same as a current day F-150 pickup. That Cadillac also got about 10.5 miles per gallon of gasoline.

In that era fuel was relatively cheap and seemingly endless in supply. There wasn’t much concern for increasing mile-age by streamlining cars. V8 engines with 4-barrel carburetors had the power to overcome the drag. One of the most recognized auto designers of that era: Enzo Ferrari, expressed it succinctly: “Aerodynamics are for people who can’t build engines …”

Today’s cars are obviously very different. Many have streamlined bodies designed to minimize drag.  Nearly all are significantly lighter than those of the mid-20th century.

So why all the discussion of old automobiles? Because the hydronics industry, at times, seems to treat the energy used to move heat from where it’s generated to where it’s needed in the building with the same attitude of the mid-20th century car designers. If the heat isn’t moving along fast enough, or the flow path has high resistance, just use a larger circulator.

Simple Multiplication

Every component that has flow passing through it dissipates head (e.g., mechanical energy) from the fluid. The exact amount of mechanical energy lost through a component, or a string of components in series, can be calculated using Formula 1.

Formula 1:

Where:
Pm = mechanical power dissipated (Watts)
0.4344 = a constant required by the units in the formula
f = flow rate (gpm)
∆P = pressure drop through the component(s) (psi)

The higher the flow rate and the higher the pressure drop, the greater the mechanical power dissipated.  Here’s an example: How much mechanical power is required to move water at 120° F at 10 gpm through 120 feet of 1-inch type M copper pipe? 

The flow rate is stated, but not the pressure drop. To get that pressure drop, you can turn to several sources, such as tables, formulas or software. I like to use our Hydronics Design Studio software for such calculations because it accurately accounts for the changes in fluid properties based on temperature, which does effect pressure drop. Putting the stated numbers into the software yields a pressure drop of 2.4 psi. The rest is easy:

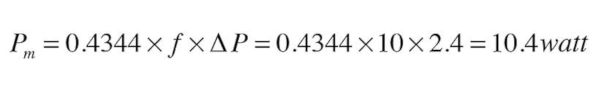
Although the units on the result are in Watts, and most of us associate Watts with electricity, this is not the electrical input power required to sustain the stated conditions. It’s the mechanical output power required by the circulator. 

We pay for the electrical energy input to the circulator, not the output power. To get the input power, we would need to know the wire-to-water efficiency of the circulator creating this condition. The efficiency is very dependent on where the circulator is operating on its pump curve. Ideally, the circulator is operating near the middle of its pump curve, where the wire to water efficiency is highest.  

If we assume a small circulator with a PSC motor is operating under favorable conditions (e.g., near the center of its pump curve), a reasonable value for wire to water efficiency is 20-25%. Let’s split the difference and assume 22.5%.

To get the electrical input power to the circulator, divide the mechanical output power (which we determined to be 10.4 Watts) by the wire-to-water efficiency of the circulator, as shown in Formula 2.

Formula 2:

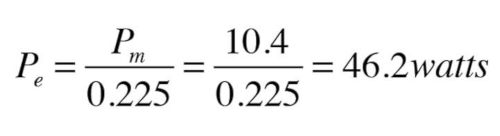
Let’s use this value as a baseline for comparison.

Do “Substitutions” Matter? 

How about if we used 1-inch PEX tubing instead of 1-inch type M copper tubing for this segment of the system, and change the circulator, if necessary, to ensure that the same 10 gpm flow is achieved? 

Changing the pipe changes the pressure drop. The Hydronics Design Studio software quickly returns a pressure drop of 6.24 psi for 120 feet of 1-inch PEX operating with water at 120° F and 10 gpm. This pushes the mechanical power re-quirement to 27.1 Watts, and the circulator input power (assuming the same 22.5 percent wire-to-water efficiency) to 120 Watts. That’s a significant increase in pumping power. It’s the result of the smaller internal diameter of 1-inch PEX (0.875”) versus that of 1-inch type M copper (1.055”).

You might be thinking I made up this example to dissuade you from using PEX rather than copper for distribution pip-ing. Not so. Instead, I’m trying to show that what might seem like an “equivalent” substitute (1-inch PEX for 1-inch cop-per) can have ramifications. In this case, it adds significant operating cost over the life of the system. 

If we assume that flow passes through the this 120 feet of piping for an average of 3,000 hours per year, and electrical energy costs $0.15 per kWh, and that electrical energy costs escalate at 2% per year, the difference in operating cost over 20 years would be $807!  That’s just for this 120 feet of 1 inch piping. You won’t pay it, but your customer will.

Moving Forward

If you design, install or manufacture hardware for hydronic systems, you should be continually thinking about ways to reduce the electrical operating cost of those systems.  

Our industry should embrace ways to reduce circulator count. One example would be eliminating “dedicated” primary loop circulators. Those that only push flow through the primary loop, and not the heat source or any load circuits. 

Another approach would be using (or creating) heat sources that don’t have high head loss heat exchangers, and thus conserve head energy. In my opinion, a “self-buffering” heat source with very low head loss is ideal. It eliminates the need for a buffer tank as well as a circulator between that tank and the heat source. This approach is likely to cost less (materials plus labor) and install faster than using a heat source combined with a separate buffer tank and all the piping, fittings, valves and circulator between them.

The use of variable speed circulators with integral speed control algorithms used in combination with valve-based zoning rather than dedicated zone circulators is another way to reduce the power demand of distribution systems.  

Figure 1 shows several of these concepts.

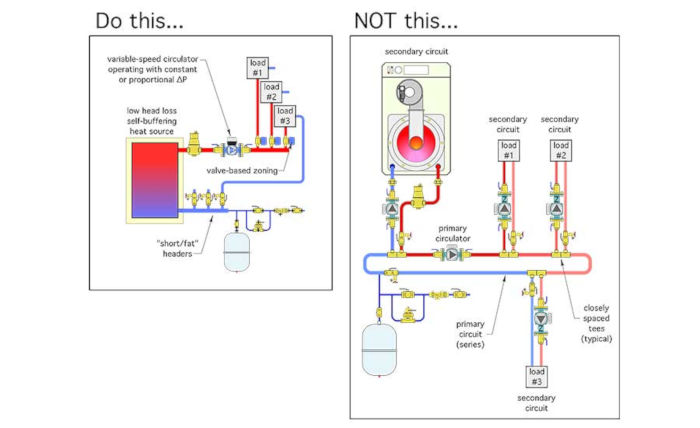
We should avoid using excessively oversized circulators and then throttling away their excess head with balancing valves. That’s analogous to driving a car around with the gas pedal pressed down farther than necessary and controlling speed with partially applied brakes.  

We should use dirt separators that don’t increase their pressure drop as internal screens load up.  

We should use glycol-based antifreeze “judiciously.” The higher the concentration of glycol, the higher the density and viscosity of the solution. The higher the ratio of viscosity divided by density, the greater the “drag” as fluid passes through the system.

If your piping layout requires a lot of 90° turns, consider using a tube bender to create larger radius bends rather than using standard 90° elbows. Larger radius bends decrease turbulence and thus decrease head energy dissipation.

We should also take a close look at heat emitters such as panel radiators, which can operate with design load ∆Ts in the range of 30-36° F rather than 20° F. The higher the ∆T the lower the required flow rate, and thus the lower the head loss for a given pipe size.

We should compare the added cost of using one size larger piping, and thus reduced head loss, against the life-cycle electrical energy savings associated with a smaller circulator.

The science of fluid dynamics has been extensively applied in many technologies where a fluid moves across a surface. Compare the planes, cars and boats of the mid-20th century to those of today. The shapes are very different, and the reduction in energy use due to decreased drag has been remarkable. 

If our charge as professionals is to improve the overall energy efficiency of the methods and materials we assemble, we shouldn’t treat the electrical energy used by our distribution systems is trivial.  Energy, as fuel or as flow, is a terrible thing to waste!