Anyone who has worked on the technical side of the hydronics industry has used terminology such as: pressure, differential pressure, head and head loss. These terms all have legitimate and specific meanings. But when some of these words get scrambled into jargon, the result can be an undefined or meaningless term. One example of such a scramble is “head pressure.”

To see why the phrase “head pressure” is not a valid technical term, it’s important to understand both words.

Let’s start with “head.” I don’t know who coined this word for use in fluid mechanics, but I do know where the concept that the word represents came from. It’s attributable to a man named Daniel Bernoulli who, in 1738, published a book entitled Hydrodynamica. That book presented a concept that is now embodied in what’s appropriately called Bernoulli’s equation. That equation is used by everyone who studies fluid motion. It explains the workings of everything from a pipeline conveying water to a city from a reservoir that’s miles away, to the shape of airplane wings. Suffice it to say that if engineers preceding us had not applied Bernoulli’s equation, our “modern” lives would be very different.

Fundamentally, Bernoulli’s equation describes the mechanical energy present in a fluid and how that energy can be transformed as the fluid changes height, pressure and speed. The “head” of a fluid refers to the total mechanical energy contained in that fluid.

In the case of a closed-loop hydronic system, head energy is added to the fluid by a circulator. Everything else the fluid flows through — piping, fittings, valves, heat emitters, etc. — removes head energy from the fluid due to the friction present between moving fluid molecules as well as between those molecules and the surfaces they come in contact with.

The strange part is that we can’t “see” energy. We can’t see it with our bare eyes, or under a microscope. Think about it — have you ever seen a Btu, or a Kilowatt•hour, or a Joule of energy? Neither have I.

Although we can’t see it directly, we can still detect when energy is added to or removed from a material. For example, consider water flowing into a boiler at 140° F and leaving that boiler at 155° F. If the piping and the boiler where transparent, the water coming out would look identical to the water going in. Yet we know there’s more thermal energy in 155° F water compared with 140° F.

The indicator of that additional thermal energy is a temperature rise. When thermal energy is added to a material (and the material doesn’t change phase between being a solid, liquid or gas), the temperature of that material increases. Conversely, when thermal energy is removed from a material, and the material remains in the same phase, its temperature goes down. Thus, a change in temperature is the evidence that thermal energy — which we can’t directly see — has been added to or removed from the material.

When it comes to head energy and pressure, there’s an analogy to the relationship between thermal energy and temperature. A decrease in pressure is the evidence that head energy has been removed from a liquid. An increase in pressure is the evidence that head energy has been added to the liquid. We can’t see head energy, but we can detect it being added or removed from a liquid by measuring changes in pressure.

In North America, the unit used to express head energy is “feet.” The word “feet” has undoubtedly caused a lot of confusion over the years. I know it confused me for a while. Why would energy be expressed in units that are commonly used for distance? Here, again, past practices have prevailed.

The unit of feet, abbreviated as ft, comes from a mathematical simplification of the units, as shown here:

ft • lb

lb

This arrangement of units would be properly stated as “foot pounds per pound.”

The unit foot pound, abbreviated as ft•lb is a valid unit of energy. As such can be converted into any other valid unit of energy. For example: 1 ft•lb = 0.0012850675 Btu.

Consider water being propelled through an operating circulator. In this situation, the arrangement of units above can be interpreted as the number of ft•lb of mechanical energy added to each pound of water passing through the circulator. Thus, a circulator that happens to be operating at say 10 feet of head is adding 10 ft•lb of mechanical energy to each pound of water passing through the circulator.

So why don’t we say it that way (e.g., the circulator is adding 10 ft•lb of mechanical energy to each lb of water passing through it)? It’s because mathematically the units of lb in the top of the fraction of cancels out with the unit of lb in the bottom of the fraction; thus, the only remaining unit is ft. It’s shorter to just state head in feet rather than fl•lb/lb.

If I had a seat at the table when this simplification became the “standard” in the industry, probably back sometime in the 1800s, my vote would have been to keep it ft•lb/lb. It’s longer, but it better expresses the concept of energy per unit weight of liquid.

The relationship: So how does one determine the amount of head energy added or removed from a fluid based on observed change in pressure? Use Formula 1:

 

Formula 1

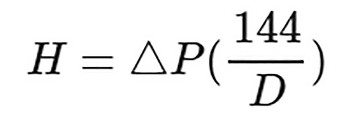
Where:

H = head (added or removed) in units of (feet)

∆P = change in pressure in units of (psi)

D = density of the fluid in units of (lb/ft³⁾

144 = a number needed for the units to work correctly

Formula 1 can be used to calculate the head energy added to the fluid when a pressure increase occurs, such as the pressure increase that can be measured across an operating circulator. The formula can also be used to calculate the head energy removed from the fluid when a pressure decrease occurs, such as across any component or group of components connected together in the circuit.

Water at 60° F has a density of about 62.4 lb/ft³. This makes the fraction of (144/62.4) equal to approximately 2.31. However, the density of water changes significantly with temperature. The density of other liquids, such as solutions of glycol-based antifreeze, is also different from that of water, and also dependent on fluid temperature.

Thus, stating that the head energy exchanged is 2.31 times the pressure change is only an approximation. It gets you in the ballpark, but the best accuracy is still attained when you use Formula 1 along with the density of the fluid. In a hydronic circuit, you can determine the density based on the average temperature of the liquid flowing through that circuit.

 

Meaningless jargon

So, back to “head pressure.” Based on what we just discussed, these two words, when paired together, are analogous to heat temperature — a term that has no meaning in our industry or any other industry.

Our industry uses plenty of jargon. For example, we might say that the output of a boiler is 80,000 Btu when what we mean is 80,000 Btu/h We might state that the electrical energy used by a small circulator is 50 Watts when what we really mean is that the power demand of the circulator is 50 Watts. We may describe a circulator operating with a head pressure of 10 feet when what we mean is a head of 10 feet.

Jargon is usually acceptable, and perhaps even a bit “admirable,” when describing hardware. For example, how many mere mortals know what is meant by a blind flange, lockshield valve, street ell or bullhead tee?

However, when learning to manage the physics that determine how the system operates, jargon often clouds understanding. That leads to uncertainty, lowered confidence and even finger crossing when making design decisions. We’ve all been there at times, and it makes us (or should make us) uncomfortable.

After reading this, some may be thinking that I’m “nitpicking” about words that most of us already sort of understand. Why not be specific and take away the words “sort of” in the previous sentence? It’s precise, professional and, ultimately, profitable.